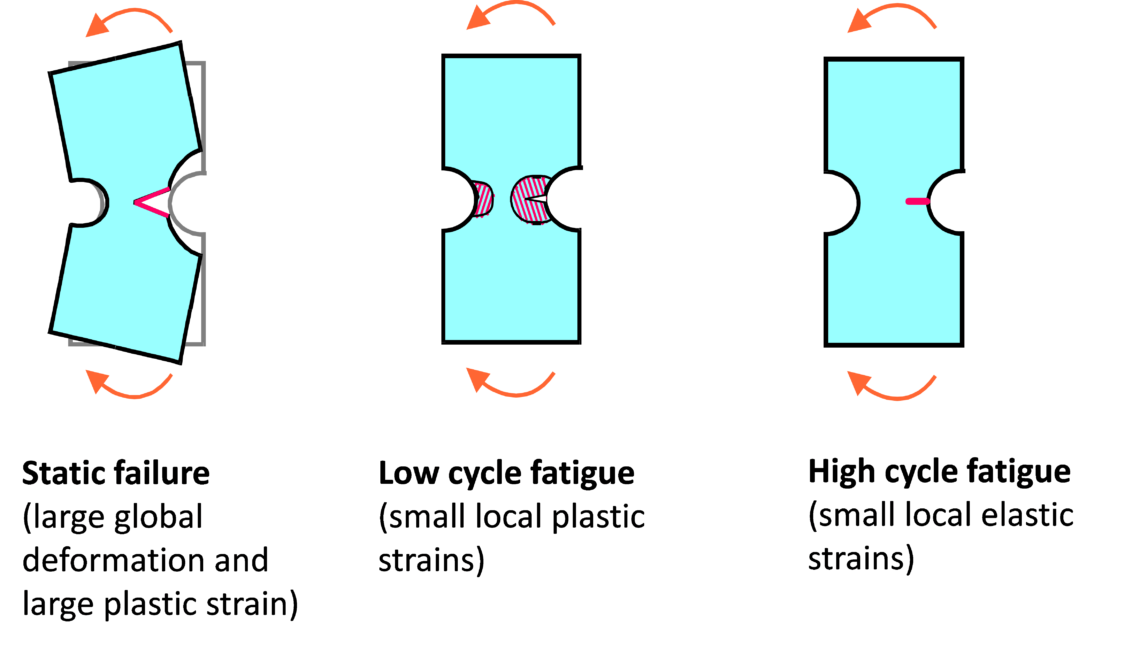
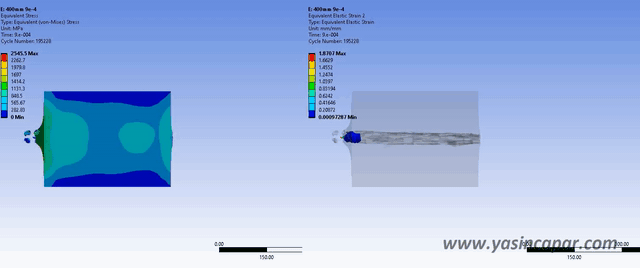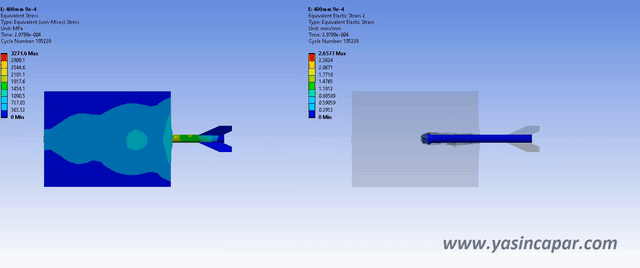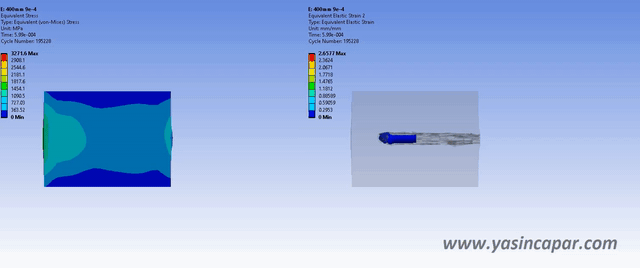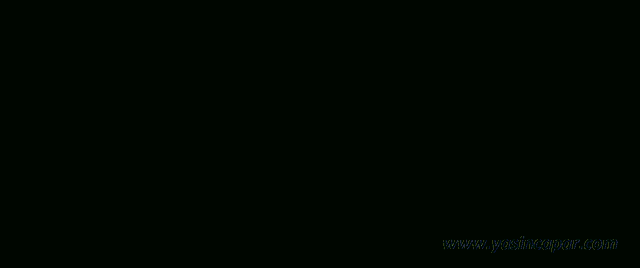
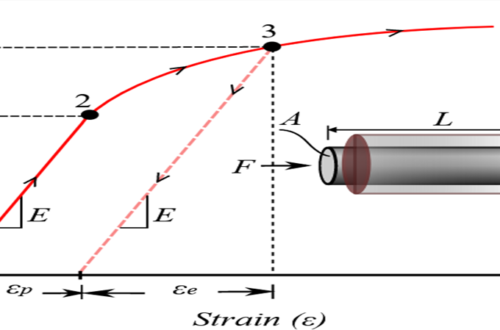
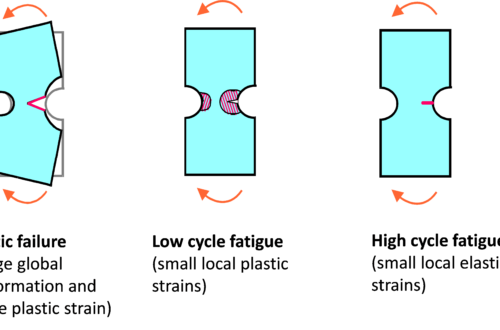
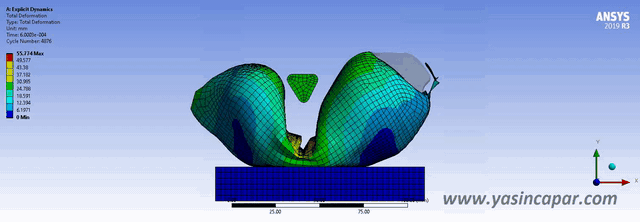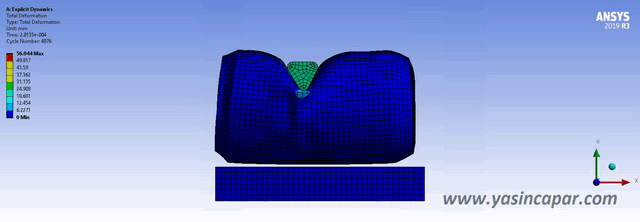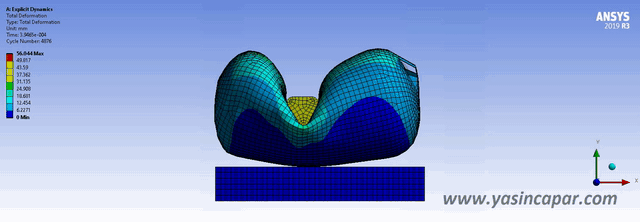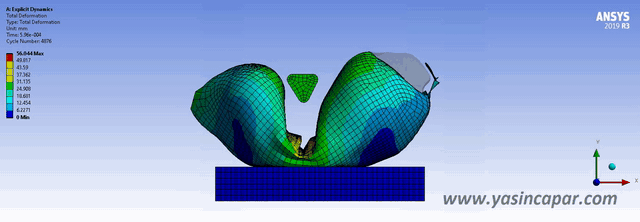
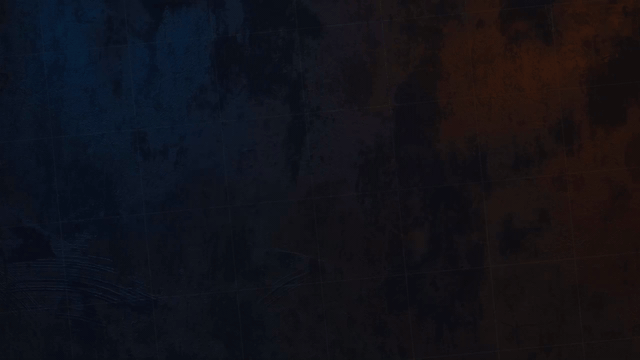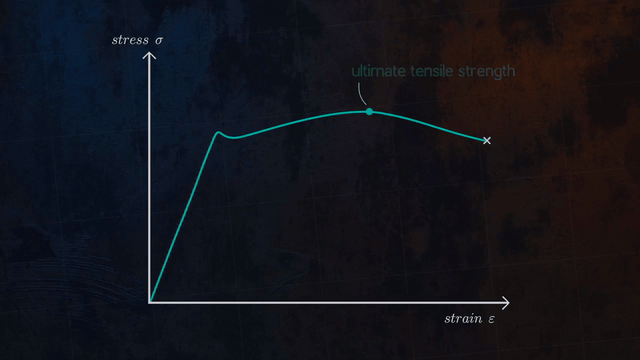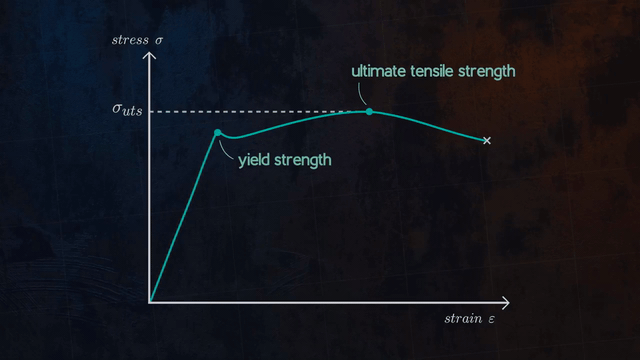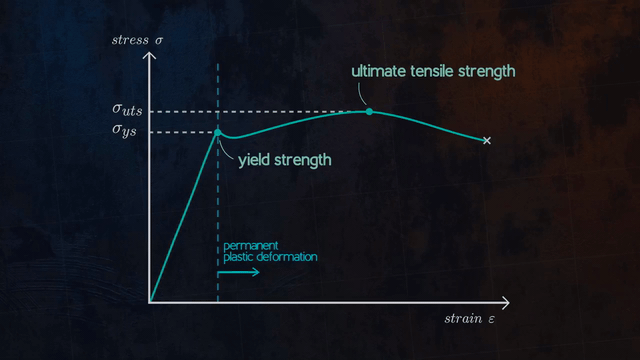In general, boundary condition loads, at a level, do not cause any damage when applied once, but when repeatedly applied, damage occurs in the form of cracks or fractures. In other words, fatigue is the changes that occur in the internal structure of the material under variable stresses. For more detail about fatigue: What is Fatigue As it is known, fatigue is a structural damage of materials under cyclic loads. There are a few main types of fatigue. Mechanical Fatigue Creep Fatigue Thermal Fatigue Thermo-Mechanical Fatigue Corrosion Fatigue Fretting Fatigue Mechanical fatigue, which is the topic of this article, could be described as damage and fracture caused of cyclic loading…
-
-
Taylor Expansion
The Taylor expansion is one of the most useful ideas in mathematics. Most functions and polynomials are generally smooth to solve. Polynomials are easier to work than almost any other sort of function, and polynomials are largely able to approximate to functions. The Taylor formula gives us an equation for the polynomial expansion for nearly every smooth function of f. Taylor Series In mathematics, the Taylor series of a function is an infinite sum of terms that are expressed in terms of the function’s derivatives at a single point. For most common functions, the function and the sum of its Taylor series are equal near this point. Taylor’s series are…
-
Plasticity
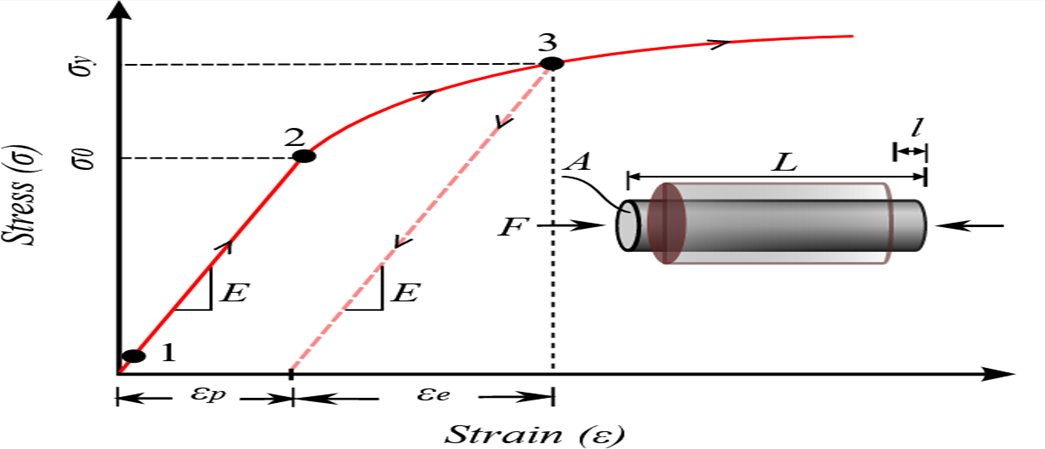
In materials science, plasticity is the ability of a solid material to undergo permanent deformation, a non-reversible change of shape in response to applied forces. For instance, a solid piece of metal being bent into a new shape displays plasticity as permanent changes occur within the material itself. In engineering, the transition from elastic behavior to plastic behavior is known as yielding. Plastic deformation is observed in most materials, especially in metals. In brittle materials such as rock, concrete, bone and so on, plasticity is caused mainly by slip at microcracks. For ductile metals, each increment of load is accompanied by a proportional increment in extension under elastic behaviour. When…
-
Material Hardening Laws
The hardening law, also known as hardening rules, describes how the yield surface changes under the plastic deformation. The hardening rule governs the change in material strength as the plastic material deformation. The change in material strength can also be thought of as a change in the geometry or position of the yielding surface. The developed yield surface is often called as the loading surface. In the perfectly plastic case, plastic deformation starts to happen when the stress reaches the yield A point during the stress is maintained there. When the stress is reduced, elastic unloading occurs. In the hardening law, once yield occurs, the stress needs to keep increasing…
-
Characteristic Length in Explicit Analysis
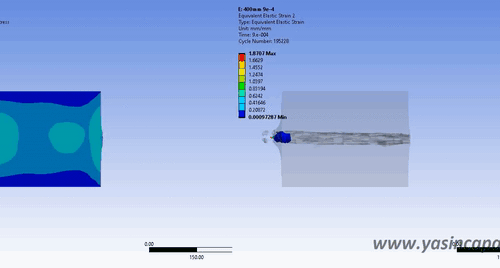
Explicit integration performs a key role in many analysis of linear and non-linear dynamics. The finite element method applies to spatial discretization in a system of ordinary differential equations to be solved, which is often done by the central difference method. Characteristic length is an important dimension that defines the scale of a physical system. Often, such a length is used as an input to a formula in order to predict some characteristics of the system, and it is usually required by the construction of a dimensionless quantity. In computational mechanics, a characteristic length is defined to force localization of a stress softening. The length is associated with an integration…
-
Engineering Stress/Strain vs True Stress/Strain
In engineering and materials science, stress–strain curve for a material gives the relationship between stress and strain. That is obtained by gradually applying load to a test coupon and measuring the deformation from tensile testing, which the stress and strain can be determined. These curves reveal many of properties of materials, such as the Young’s modulus, the yield strength, the ultimate tensile strength and so on. Stress-strain curve for material is plotted by elongating the sample and recording the stress variation with strain until the sample fractures. The strain is set to horizontal axis and stress is set to vertical axis. It is often assumed that the cross-section area of…
-
Implicit vs Explicit Approach in FEM
Explicit and implicit methods are used in numerical analysis for obtaining numerical approximations to solve solutions of time-dependent ordinary and partial differential equations which require computer simulations of physical processes. Explicit methods calculate the state of a system at a later time from the state of the system at the current time, while implicit methods find a solution by solving an equation involving both the current state of the system and the later one. For all nonlinear and dynamic analyses, incremental loads are needed. Commonly, ‘implicit’ and ‘explicit’ methods are used to solve these problems. In static analysis, there is no effect of mass (inertia) or damping. In dynamic analysis,…
-
The Newton-Raphson Method
In numerical analysis, the Newton–Raphson method (also known as Newton’s method), named after Isaac Newton and Joseph Raphson, is a method for finding successively and quickly better approximations for the roots of real-valued functions. The Newton-Raphson method is a powerful technique for solving equations numerically. It uses the idea that a continuous and differentiable function can be approximated by a straight line tangent to it. The idea starts with an initial guess which is reasonably close to the true root, then to approximate the function by its tangent line, and to compute the x-intercept of this tangent line by elementary algebra. This x-intercept will typically be a better approximation to…
-
Nonlinearities in FEM
A linear FEA study simulates reality only when a number of restricting conditions are defined. These include that all deflections are so small and, that stress and strains induced by loads remain small enough to let their linear relationship (Hooke’s law) hold. A linear analysis could miss the target because it fails to detect the geometry’s unstable regions. In these regions, even a small change in load amplitude could lead to considerable different deformations. Linear finite element method simplifies a lot of things. For instance, the material will never yield resulting in unrealistically high stresses in model. Also, you might not predict failing because nonlinear geometry is not considered. FEA…
-
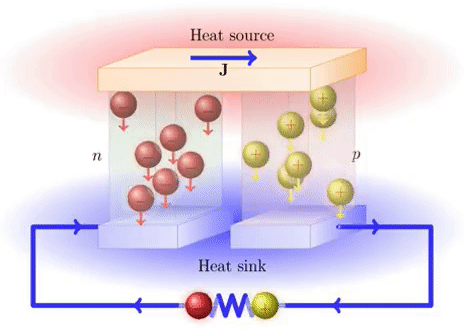
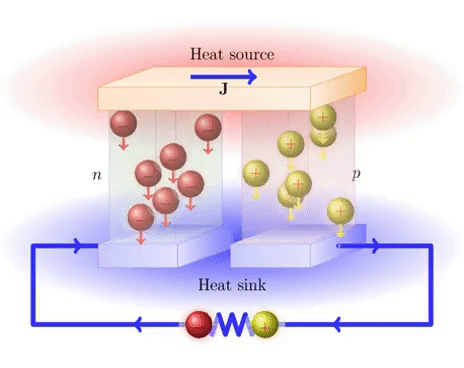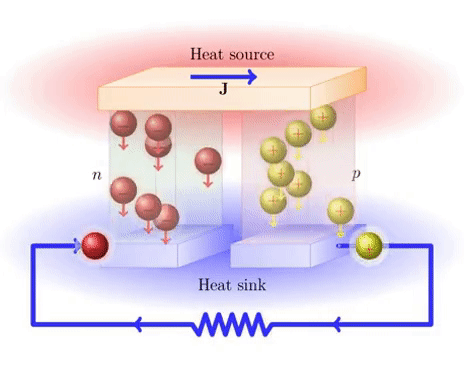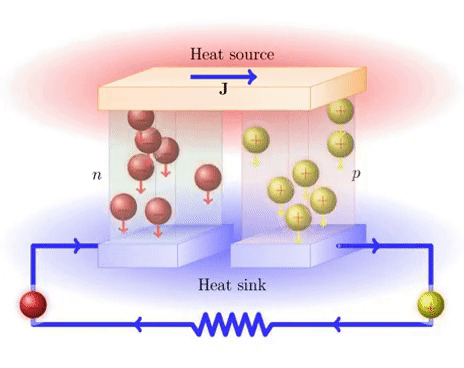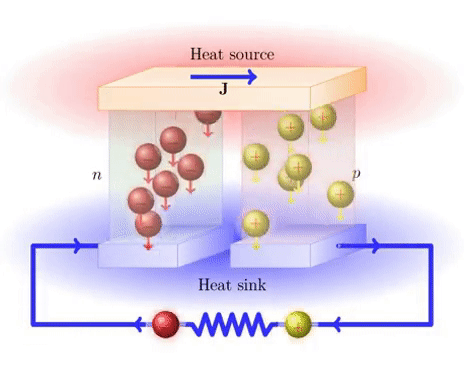
Thermoelectric Effect
Theoretical Framework The circuit, in which both heat and electrical factors coexist, is called a thermoelectric circuit, and a system working with this circuit is called a thermoelectric system. Thermoelectric module consists of n and p type materials. Generally, Bi2Te3 is used as n type material and Bi0.1Sb1.9Te3 is used as p type material. Thermoelectric coolers consist of a large number of thermocouples electrically arranged in series and thermally parallel. Thermoelectric cooler modules are generally produced by placing an equal number of n and p type material pairs (Image 1). In the building area of the figure, the electric current moves through the lower and upper layers of all n…